Research nteintro
Negative Thermal Expansion Materials
I have a long-standing interest in negative thermal expansion (NTE) materials – that is materials which contract when heated. This is the opposite behaviour to the majority of materials and leads to engineering, photonic, electronic or structural applications. If, for example, one were to mix an NTE material with a “normal” material which expands on heating one could envisage making a zero expansion composite.
While there are a variety of mechanisms that can lead to negative thermal expansion, we’ve concentrated mainly on phonon-driven contraction in inorganic framework materials. The basic idea behind NTE in these materials can be appreciated relatively easily. If we consider a simple isolated M-O-M linkage, we can imagine longitudinal M-O vibrations which will tend to expand the M-O and therefore M-M distnace on heating (the asymmetry of typical interatomic potentials leads to to bonds getting longer as higher vibrational states are populated). However we can also imagine transverse M-O-M vibrations. Provided M-O distances don’t increase too rapidly with temperature these transverse vibrations will tend to pull M’s closer together as oxygens move sideways and can contribute to NTE. These modes are said to have negative Gruneisen parameters (their frequency decreases as volume decreases).
Some framework materials display what are called “rigid unit modes” (RUMs) which are distortions in which the coordination polyhedra which make up the framework can move without internal distortion. As such these tend to be low energy distortions. Many of them involve transverse movement of oxygen atoms linking the polyhedra, have negative Gruneisen parameters, and are the kinds of modes that lead to negative thermal expansion.
The rest of the page contains some of our early animations which summarise these ideas. There are now a variety of excellent reviews on NTE which explain the ideas in more detail.
Rigid Unit Modes
The animation below shows how a network of corner sharing MO4 squares can distort without the M-O bond lengths or O-M-O bond angles changing. These “rigid unit modes” are therefore often low energy distortions of framework materials. The transverse motion of oxygen atoms means they will have a negative Gruneisen parameter and contribute to NTE when populated. This is a 2D analogue of a perovskite- or ReO3-like framework.
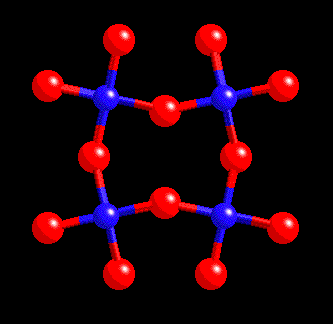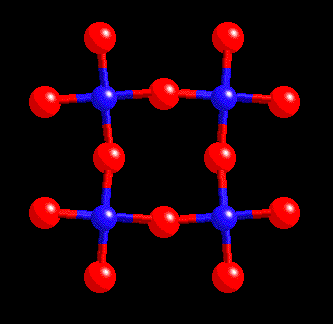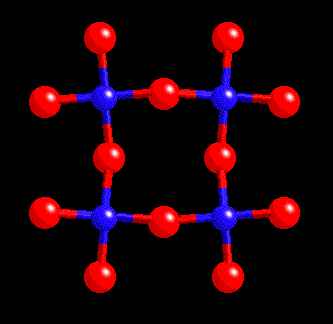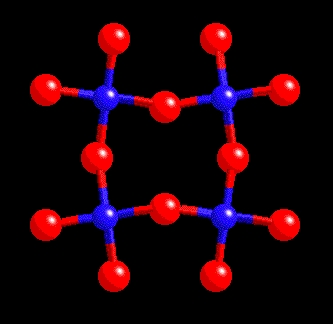
ZrW2O8
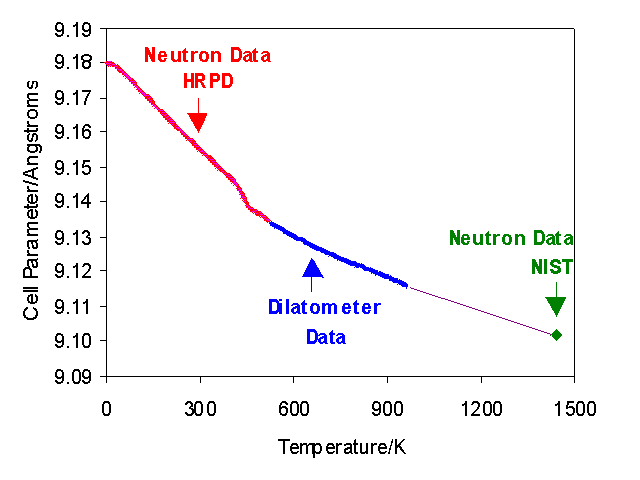
The animations below show the type of flexibility exhibited by ZrW2O8 which underpins its NTE. It’s a 3D analogue of the 2D animation above and can occur without the green ZrO6 octahedra or the red WO4 tetrahedra distorting. The most recent theoretical studies have shown that ZrW2O8 has a large number of low energy negative Gruneisen parameter modes which contribute to its NTE.

Other NTE Materials
We’ve also worked on other inorganic frameworks such as the AM2O7 and A2(MO4)3 families where NTE and phase transitions involving polyhedral tilting are intimately linked. We also described colossal NTE in frameworks such as Ag3[Co(CN)6] and super-colossal unixial NTE in the organic solid CA-Pyz as well as controllable zero thermal expansion over a wide temperature range in SnMo2O8 derivatives.
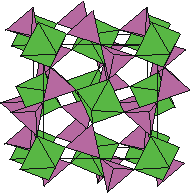
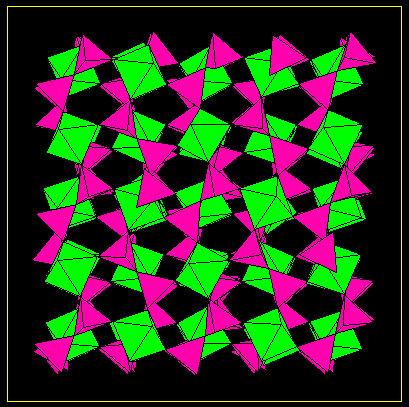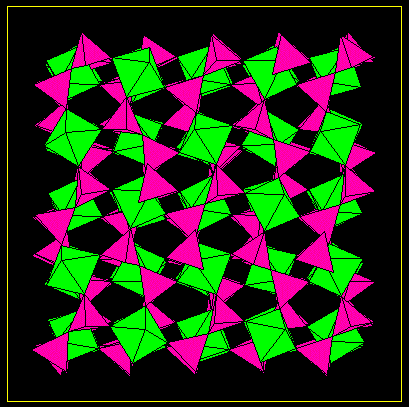
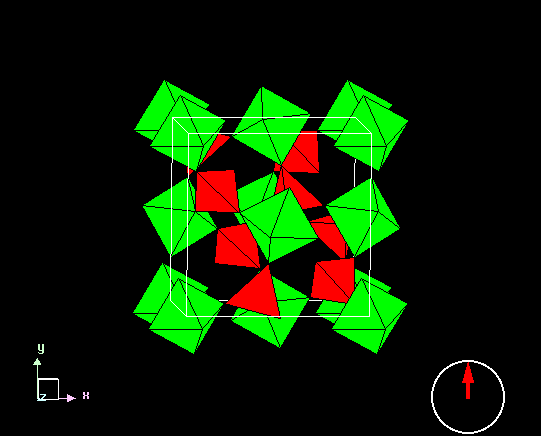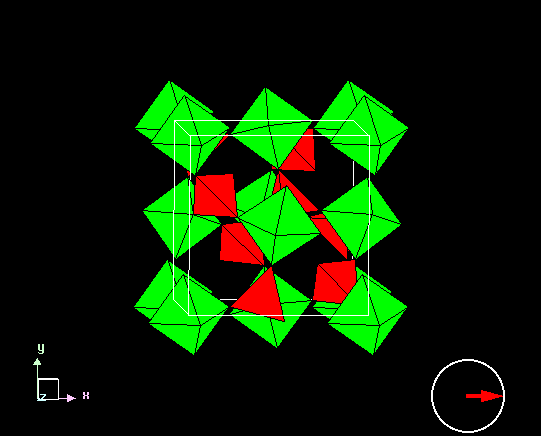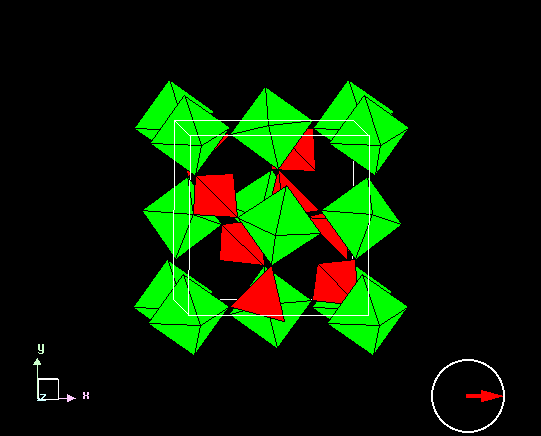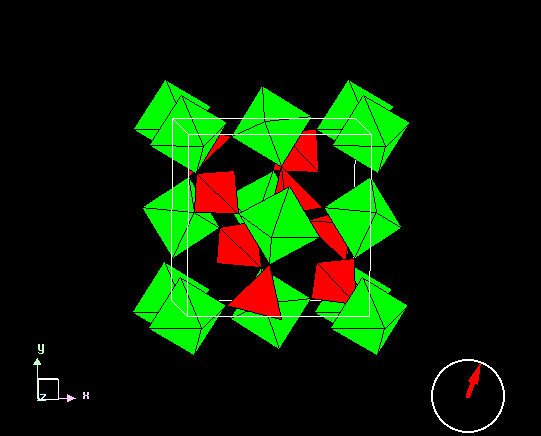
ZrV2O7 is structurally related to ZrW2O8. It displays negative thermal expansion at high (>102 C) temperatures. Below this temperature it undergoes phase transitions to an incommensurate structure, then to a 3x3x3 cubic superstructure. The superstructure contains 108 octahedra and 216 tetrahedra in each unit cell. There is more than one way in which these units can be arranged in the structure. This degeneracy is thought to be linked to the unusual thermal expansion properties.
The simple cubic cell with green ZrO6 octahedra and pink VO4 tetrahedra is shown below. The lower picture shows possible low energy arrangements of polyhedra within the unit cell of ZrV2O7. Both these arrangements contain minimally distorted ZrO6 octahedra and VO4 tetrahedra. The yellow box is the true 26.3 Angstrom cubic cell.